Last summer I worked with my maths faculty to create our own tailored Mathematics Mastery scheme of work, for year 7 specifically. We looked at different scheme of work overviews, from different schools, and compiled our own. This year it has been really interesting delivering the teaching and learning of this project.
In this blog post I will share the interesting take I took in teaching my bottom Y7 set students the double connection between identifying the number of sides of each 2D regular shape, and identifying the name of a regular 2D shape by stating the number of sides of the shape in question.
I used a rhythm mnemonic which was inspired by a visit to Michaela Community School; as a technique used to embed factual knowledge in students’ long term memory.
So here it goes:
Triangle, Triangle, Triangle (Hold three fingers each time)
Square, (Hold four fingers)
Rectangle, (Draw a rectangle in the air using two hands)
Pentagon, (Hold five fingers on one hand like a star)
Hexagon, (Hold six fingers)
Octagon, (wave both arms like an octopus)
Nonagon, (Hold nine fingers)
Decagon, (Hold ten fingers)
And all the way around like a circle (Draw a circle using two hands) – The intention is to avoid the misconception that a circle has 0 sides when it has 1 curved side.
https://www.youtube.com/watch?v=ieDddH-NvwI
Initially, students enjoyed the experience of learning the mnemonic, and I asked my TA and Deputy Head – who teaches the same group (science) –to actively stop the girls in the corridor, in order for them to practise it. The continual repetition of students practising the mnemonic, to a rhythm and in using their hands, allowed these students to identify the number of sides for each regular 2D shape.
These results were identified when I was undertaking a few mini-whiteboard quizzes (AfL) in our lessons. I could see students repeating the mnemonic in their heads, and indeed using their fingers. However, through continual repetition, over two weeks, students slowly started transitioning away from using the mnemonic to automatically identifying that a pentagon has five sides. They were able to identify that a square has 4 equal sides, and that a rectangle does not, because of the act of drawing the shape out in the mnemonic.
After two weeks students were automatically answering the following questions in 3 – 5 seconds; whereas before they needed 15 – 25 seconds of thinking time. Once students were able to identify the number of sides for the 2D regular shape in question I started asking them to state the name of the regular 2D shape given the number of sides that shape has. This is incredibly important. Students need to draw the link that an octagon (visual image as well as the text) has 8 sides, and that if a shape has 8 sides it is identified as an octagon. That dual train of thought must be connected to ensure that students had successfully achieved the learning objective: to be able to identify the number of sides for a selection of 2D regular shapes. This was something I invented off the cuff, when I realised that students were not successfully retaining the number of sides each 2D shapes from the way I initially planned my lesson. It worked well. The effectiveness was in the consistency of practising the rhythm mnemonic to ensure that the mnemonic and the facts from that mnemonic are embedded as factual knowledge in students’ long term memory. We have chanted this mnemonic over a hundred times. We are not bored of it. We love it because students can see the success for themselves. It is a learning tool to aid the initial build-up of basic factual knowledge. I have attached a video of my students demonstrating the mnemonic on my twitter account (@naveenfrizvi). I hope it is useful to you.
https://www.youtube.com/watch?v=Zp8K5bMd6nY





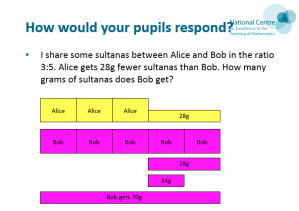

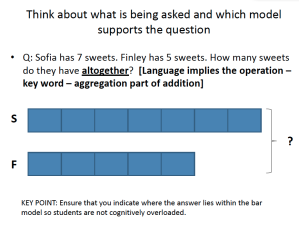
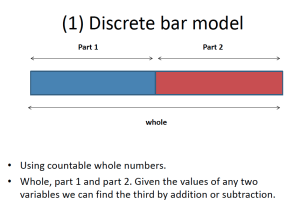
 (3) Multiplicative comparison models
(3) Multiplicative comparison models 
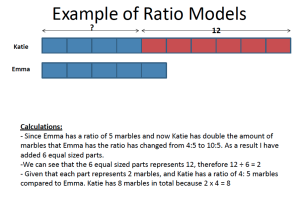
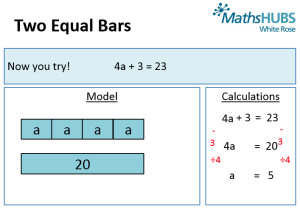
 Now it is your turn to try a question that Sue asked us keen teachers to try? I shall reveal the answer next week.
Now it is your turn to try a question that Sue asked us keen teachers to try? I shall reveal the answer next week. 
Recent Comments